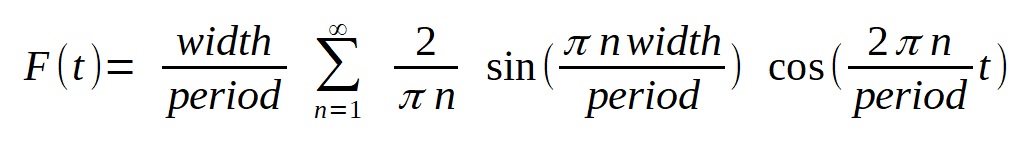1. Ultrashort Pulse
2. Pulse Wave
Every function
can be represented by the summation of individual
frequency components
, the so called
Fourier Series
. With a set of given single frequencies a new time signal can be generated.
On the other hand by
sampling
a time signal with respect to its frequency components, the
source frequencies
of the time signal can be revealed. This process is known under the term
Fourier Transformation
and widely used in engineering and science.
The
interactive visualization
on this pages exemplifies the Fourier Transformation and its characteristic parameters. The Graphs show; the resulting or starting time signal (top), the single frequency terms (bottom-left) and the spectral representation of the frequency components (bottom-right). The blue lines in the spectrum indicate the individual frequencies (
Delta Dirac Functions
); the connecting dark line is (spline-) interpolated.
The Fourier Series for generating a rectangular function or pulse train is shown below. The parameters
Pulse-Period T
,
Pulse-Width
and
n-Number of Frequencies
can be set by the sliders above.